Abstract
This algorithm generates all subsets of n with k elements.
The Excel function COMBIN(n, k) shows the count of all combinations, but the algorithm presented here lists all combinations individually so that you can examine them if necessary. The program is fast - you can compare to the formula solutions you can find below under Other Links.
Example
Input:
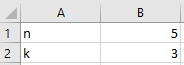
Output:
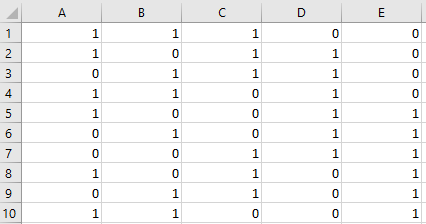
Literature
Reingold, Nievergelt, Deo: Combinatorial Algorithms, 1977, Algorithm 5.9, p. 186, ISBN 0-13-152447-X
Other Links
(External link!) Efficient approach to generate list of combinations with no repetition
Appendix – Combinations_with_k_subsets_of_n Code
Please read my Disclaimer.
Option Explicit
Public r As Long 'Output row
'Generates all combinations of array n with subsets k.
'See Reingold, Nievergelt, Deo: Combinatorial Algorithms, 1977, Algorithm 5.9, p. 186, ISBN 0-13-152447-X
'Version 0.2 12-Jul-2024
Sub Combinations_with_k_subsets_of_n(n As Long, k As Long)
Dim i As Long, j As Long, m As Long, t As Long, u As Long
With Application.WorksheetFunction
wsO.Cells.ClearContents
ReDim g(1 To n + 1) As Long
ReDim tau(1 To n + 1) As Long
ReDim res(1 To .Combin(n, k), 1 To n) As Variant
r = 1
For j = 1 To k
g(j) = 1
tau(j) = j + 1
Next j
For j = k + 1 To n + 1
g(j) = 0
tau(j) = j + 1
Next j
t = k
tau(1) = k + 1
i = 0
Do While i <> n + 1
'Call Visit(g) instead of the next 4 rows if you need to analyze g().
For u = 1 To UBound(g) - 1
res(r, u) = g(u)
Next u
r = r + 1
i = tau(1)
tau(1) = tau(i)
tau(i) = i + 1
If g(i) = 1 Then
If t <> 0 Then
g(t) = 1 - g(t)
Else
g(i - 1) = 1 - g(i - 1)
End If
t = t + 1
Else
If t <> 1 Then
g(t - 1) = 1 - g(t - 1)
Else
g(i - 1) = 1 - g(i - 1)
End If
t = t - 1
End If
g(i) = 1 - g(i)
If t = i - 1 Or t = 0 Then
t = t + 1
Else
t = t - g(i - 1)
tau(i - 1) = tau(1)
If t = 0 Then
tau(1) = i - 1
Else
tau(1) = t + 1
End If
End If
Loop
Range(wsO.Cells(1, 1), wsO.Cells(r - 1, n)) = res
End With
End Sub
Sub Visit(g As Variant)
'Print current permutation in immediate window and on sheet Output.
'You can analyze the permutation or do other things as well.
Dim i As Long
For i = 1 To UBound(g) - 1
wsO.Cells(r, i) = g(i)
Debug.Print g(i);
Next i
Debug.Print
r = r + 1
End Sub
Sub test()
Debug.Print Now
Call Combinations_with_k_subsets_of_n(5, 3)
Debug.Print Now
End Sub
Download
Please read my Disclaimer.
combinations_with_k_subsets_of_n.xlsm [26 KB Excel file, open and use at your own risk]